Balmer series and long-time dynamics

Balmer series, or Balmer lines, are the visible part of the spectrum corresponding to the electron transitions in Hydrogen atom.

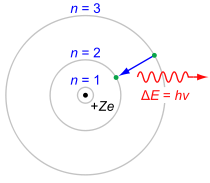
More precisely, Balmer series correspond to jumps from d, e, f, ... energy levels (n3, with n being the principal quantum number) onto the p energy levels (n=2). Only four of these spectral lines are visible; others are ultraviolet.

Bohr's model (both pictures are from Balmer series article at Wikipedia).

Transitions onto s energy levels (n=1) are accompanied by ultraviolet photons. Transitions onto orbits d and above (n3) are accopmanied by infrared photons.

The sharp spectral lines in the emission spectrum of excited Hydrogen atoms suggest that as time goes to plus or minus infinity the electron is in the state corresponding to one of the Bohr orbits; not to a superposition of such states.

The Quantum Mechanical description of this is in postulating that the electron lives on the Bohr orbit and jumps from one orbit onto another. A more sophisticated explanation is to say that observing the spectral lines corresponds to measuring the energy and hence the system ends up in an eigenstate of the energy operator (not in a superposition of such states). This explanation makes little sense mathematically.

In fact, the absence of such a superposition in the long-time asymptotics can not be explained in the framework of the linear Schrödinger (or Dirac) equation with the Coulomb potential, or, for that matter, in the framework of any linear model. In turn, this suggests that the adequate description of the dynamics of the electron needs to take into account the nonlinear self-interaction of the electron with its own electromagnetic field.

In this dynamical description, the Schröodinger eigenstates are the approximations of certain nonlinear eigenfunctions which are to be interpreted as the points of the attractor of the system.